A different kind of double pendulum consists of an extra arm of length 
(Fig. 2). This system has a slightly different Lagrangian from that of the usual double pendulum, but still has the same two degrees of freedom and so the same method of solution can be used. Expressions for the elements of the matrices 
and 
, and components of the vector 
should be substituted by those given below.
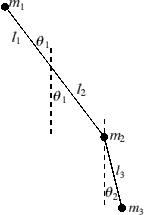
Figure 1: The modified double pendulum set-up. The lengths 
, 
and 
, and the masses 
, 
and 
are fixed. The system has the degrees of freedom 
and 
.
The components of the Lagrangian for this modified system are,
The relevant transformations are,
Inserting Eqs. (7.3) to (7.8) into Eq. (7.2) (and simplifying), the Lagrangian becomes,
The following, for the modified system, replace Eqs. (5.1) to (5.10),
